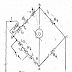
Heaviside Mutual Inductance Bridge.

  Heaviside Mutual Inductance Bridge.

This bridge uses known self-inductance to measure mutual inductance. The same bridge slightly modified by Campbell is used to measure self-inductance in terms of known mutual inductance.

M = unknown mutual inductance,

L1 = self-inductance of secondary of mutual inductance,

L2 = known self-inductance,

and R1 , R2 ,R3 , R4 = non-inductive resistors.

At balance voltage drop across between b and c must equal to the voltage drop across between d and c. Also, the voltage drop across ahc must equal to the voltage drop across adc. Thus we have the following equations at balance.

                    I1R3 = I2.R4

               (I1+I2)(jwM)+I1(R1+R3+jwL1) = I2(R2+R4+jwL2)

Solving them we get:

                R1.R4 = R2.R3

and           M = (R3.L2 - R4.L1)/(R4+R3)

if R3 = R4    ,  we get,  M = (L2 - L1)/2

                           and     R1 = R2

This method can also ussed for measurement of self inductance. Suppose if we have to determine L2 then

 we can write ,                L2 = (M(R3+R4) + R4.L1 )/R3

                                             = M ( 1 + R4/R3) + ( R4/R3 ).L1

                        and R2 = R1.(R4/R3)

In case             R4 = R3, we have

                        L2 = 2M +L1

 and                 R2 = R1